These resources will be removed by end of Summer Term 2025.
Switch to our new teaching resources now - designed by teachers and leading subject experts, and tested in classrooms.
These resources were created for remote use during the pandemic and are not designed for classroom teaching.
Lesson details
Key learning points
- In this lesson, we will learn how to determine the shape, roots and y-intercept of a graph from an equation.
Licence
This content is made available by Oak National Academy Limited and its partners and licensed under Oak’s terms & conditions (Collection 1), except where otherwise stated.
5 Questions
Q1.
A quadratic equation must have two solutions. True or false?
True
Q2.
what are the solutions to the quadratic equation in question a?
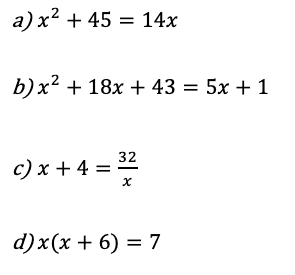
x = -3 or x = -15
x = -5 or x = -9
x= - 15 and x = -3
Q3.
what are the solutions to the quadratic equation in question b?
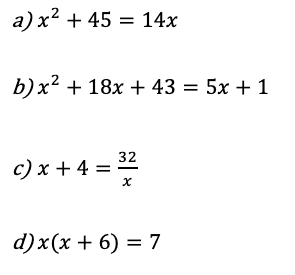
x = -14 or x = -3
x = 14 or x = 3
x = 6 or x = 7
Q4.
what are the solutions to the quadratic equation in question c?
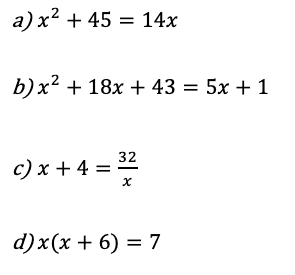
x = -3 or x = 7
x = -4 or x = 4
x= 3 and x = -7
Q5.
what are the solutions to the quadratic equation in question d?
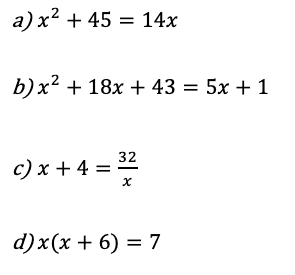
x = -1 or x = 7
x = 0 or x = -6
x = 0 or x = 6
5 Questions
Q1.
What are the roots of the quadratic equation?
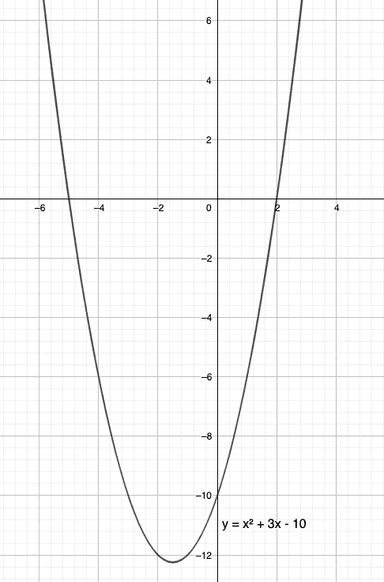
x = -1.5
x = 2 and x = -4.5
x= -10
Q2.
What is the y-intercept of the quadratic equation?
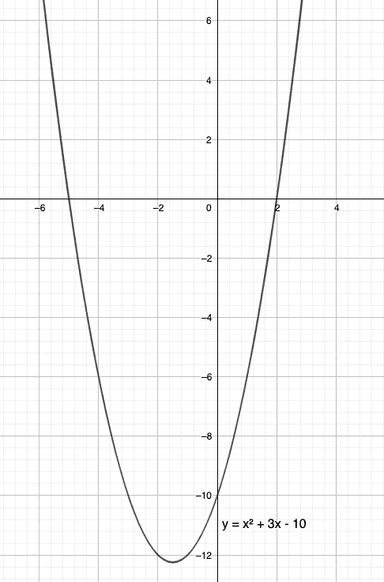
(-10,0)
(0,-10)
(10,0)
Q3.
What is the y-intercept?
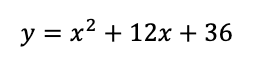
(0,-36)
(0,-6)
(0,6)
Q4.
What are the roots of this equation?
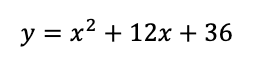
x = -4 and x = -9
x = 6
x= 4 and x = 9
Q5.
What are the roots of this equation?
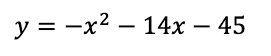
x = -4 and x = -10
x = 4 and x = 10
x= 5 and x = 9

