Myths about teaching can hold you back


- Year 11•
- Foundation
Problem solving with further surface area and volume
I can use my enhanced knowledge of surface area and volume to solve problems.


- Year 11•
- Foundation
Problem solving with further surface area and volume
I can use my enhanced knowledge of surface area and volume to solve problems.
These resources will be removed by end of Summer Term 2025.
Switch to our new teaching resources now - designed by teachers and leading subject experts, and tested in classrooms.
These resources were created for remote use during the pandemic and are not designed for classroom teaching.
Lesson details
Key learning points
- The surface area of many solids can be calculated by a known method.
- The volume of many solids can be calculated by a known method.
- Writing an algebraic statement about surface area/volume can be done from a diagram.
Keywords
Prism - A prism is a polyhedron with a base that is a polygon and a parallel opposite face that is identical. The corresponding edges of the two polygons are joined by parallelograms.
Cylinder - A cylinder is a 3D shape with a base that is a circle and a parallel opposite face that is identical. A cross-section of a cylinder made parallel to the base will be congruent to the base.
Surface area - The surface area is the total area of all the surfaces of a closed 3D shape. The surfaces include all faces and any curved surfaces.
Volume - Volume is the amount of space occupied by a closed 3D shape.
Common misconception
Pupils may confuse whether they need to calculate the volume or surface area of a 3D shape, if not told specifically to do so in a problem.
Use the context to decide which calculation is needed. If the question refers to packaging or painting the shape, a surface area calculation is needed.
To help you plan your year 11 maths lesson on: Problem solving with further surface area and volume, download all teaching resources for free and adapt to suit your pupils' needs...
To help you plan your year 11 maths lesson on: Problem solving with further surface area and volume, download all teaching resources for free and adapt to suit your pupils' needs.
The starter quiz will activate and check your pupils' prior knowledge, with versions available both with and without answers in PDF format.
We use learning cycles to break down learning into key concepts or ideas linked to the learning outcome. Each learning cycle features explanations with checks for understanding and practice tasks with feedback. All of this is found in our slide decks, ready for you to download and edit. The practice tasks are also available as printable worksheets and some lessons have additional materials with extra material you might need for teaching the lesson.
The assessment exit quiz will test your pupils' understanding of the key learning points.
Our video is a tool for planning, showing how other teachers might teach the lesson, offering helpful tips, modelled explanations and inspiration for your own delivery in the classroom. Plus, you can set it as homework or revision for pupils and keep their learning on track by sharing an online pupil version of this lesson.
Explore more key stage 4 maths lessons from the 2D and 3D shape: surface area and volume (pyramids, spheres and cones) unit, dive into the full secondary maths curriculum, or learn more about lesson planning.

Licence
Prior knowledge starter quiz
6 Questions
Q1.The amount of space occupied by a closed 3D shape is called the __________ of the shape.
Q2.The diagram shows a composite solid constructed from two congruent cuboids. All lengths given are in centimetres. Which of these calculations give the total volume of the solid?
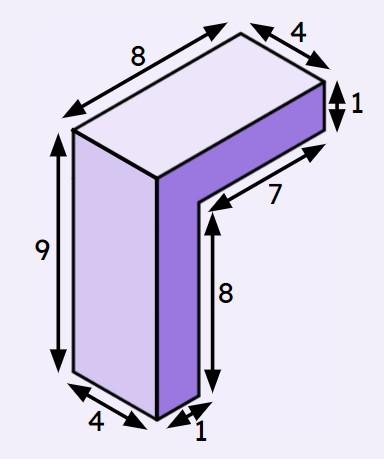
Q3.This composite solid is constructed from two cuboids. All lengths given are in millimetres. The total volume of the solid is mm³.
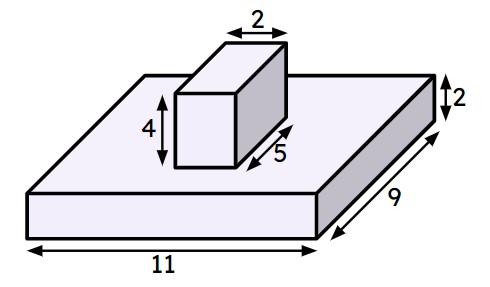
Q4.This composite solid is constructed by placing a hemisphere with diameter 8 cm on top of a cuboid. Find the volume of the solid. Give your answer to the nearest cubic centimetre.
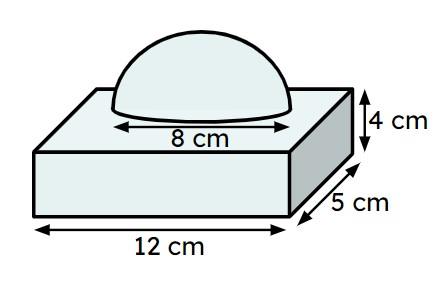
Q5.This composite solid is constructed with a cylinder and a hemisphere. Each have a diameter of 10 cm. The volume of the solid is cm³ (correct to 4 significant figures).

Q6.This composite solid is constructed with a cone and a hemisphere. The volume of the solid, in terms of 𝜋, is 𝜋 cm³.
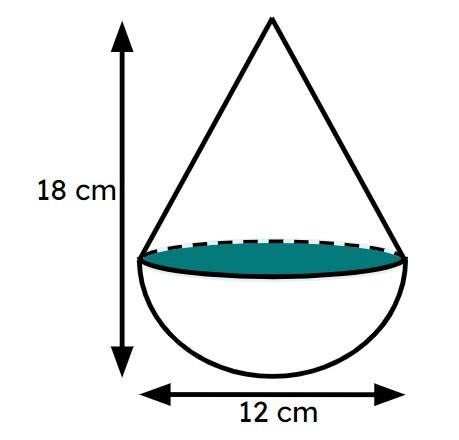
Assessment exit quiz
6 Questions
Q1.Name a 3D shape that has exactly one curved surface and no flat surfaces.
Q2.Starting with the cuboid with the smallest volume, put these cuboids into order of size according to their volumes.
Q3.Starting with the cuboid with the smallest surface area, put these cuboids into order of size according to their surface areas.
Q4.In this cuboid, the depth is $$k$$ cm. The lengths of the depth to the height to the width of the cuboid can be written in the ratio 1 : 2 : 4. Select an expression for the volume of the cuboid.
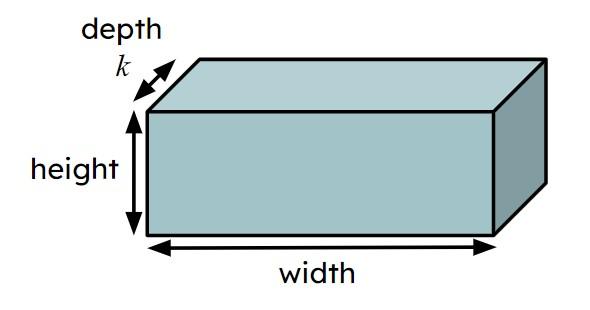
Q5.In this cuboid, the depth is $$k$$ cm. The lengths of the depth to the height to the width of the cuboid can be written in the ratio 1 : 2 : 4. Find an expression for the surface area of the cuboid.


